-
I repurposed a previous pixel drawing for today’s pixel drawing for the Divoom community. The little guy is supposed to represent a nasty germ. We’ll see how it’s received. I like it.

-
Sometimes a piece is just not worth sharing.

I made it for the Divoom community, even posted it on there, but soon deleted it, because it was not making me feel happy with it.
-
The @pixeldailies.bsky.social theme for 23 March 2025 was #jar #pixel_dailies

-
I used this image as a reference for my contribution to March of Robots 2025. Though I didn’t design the robot, I did the color palette and animation.
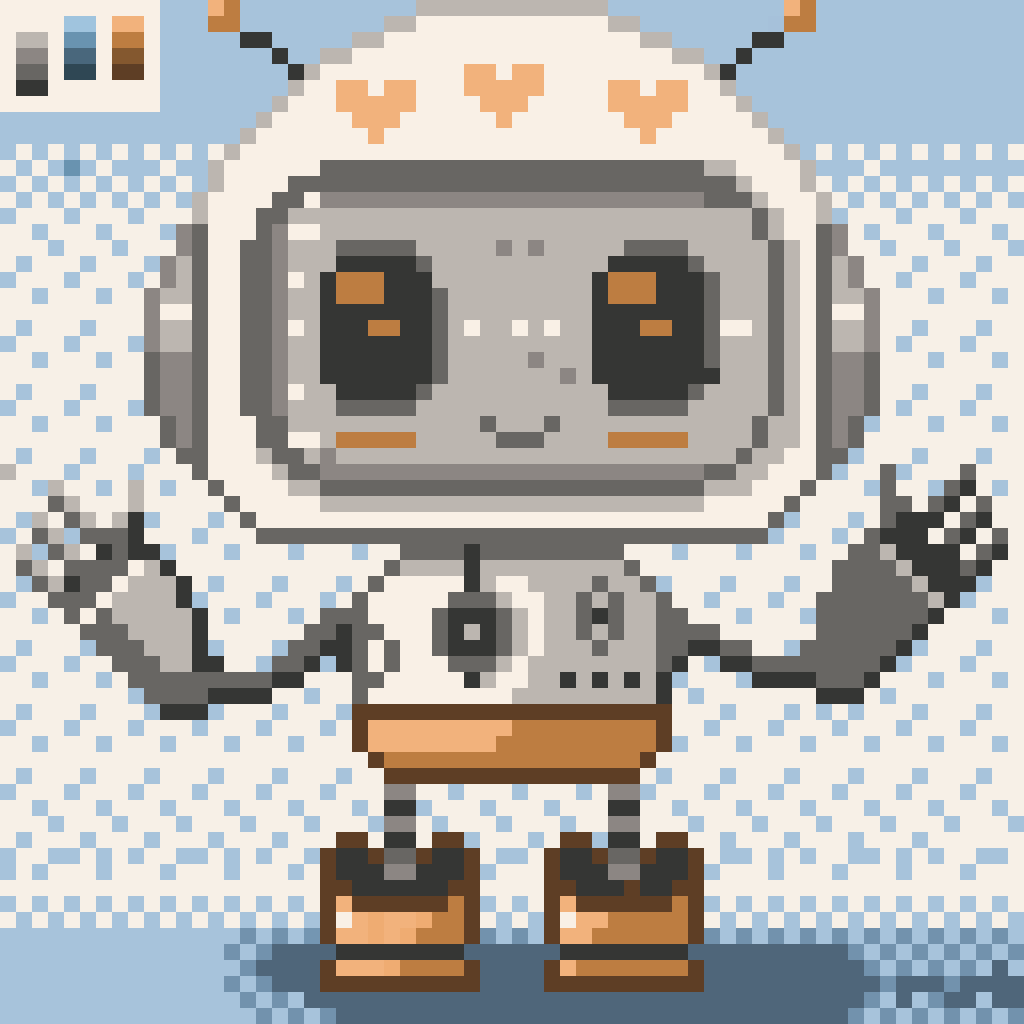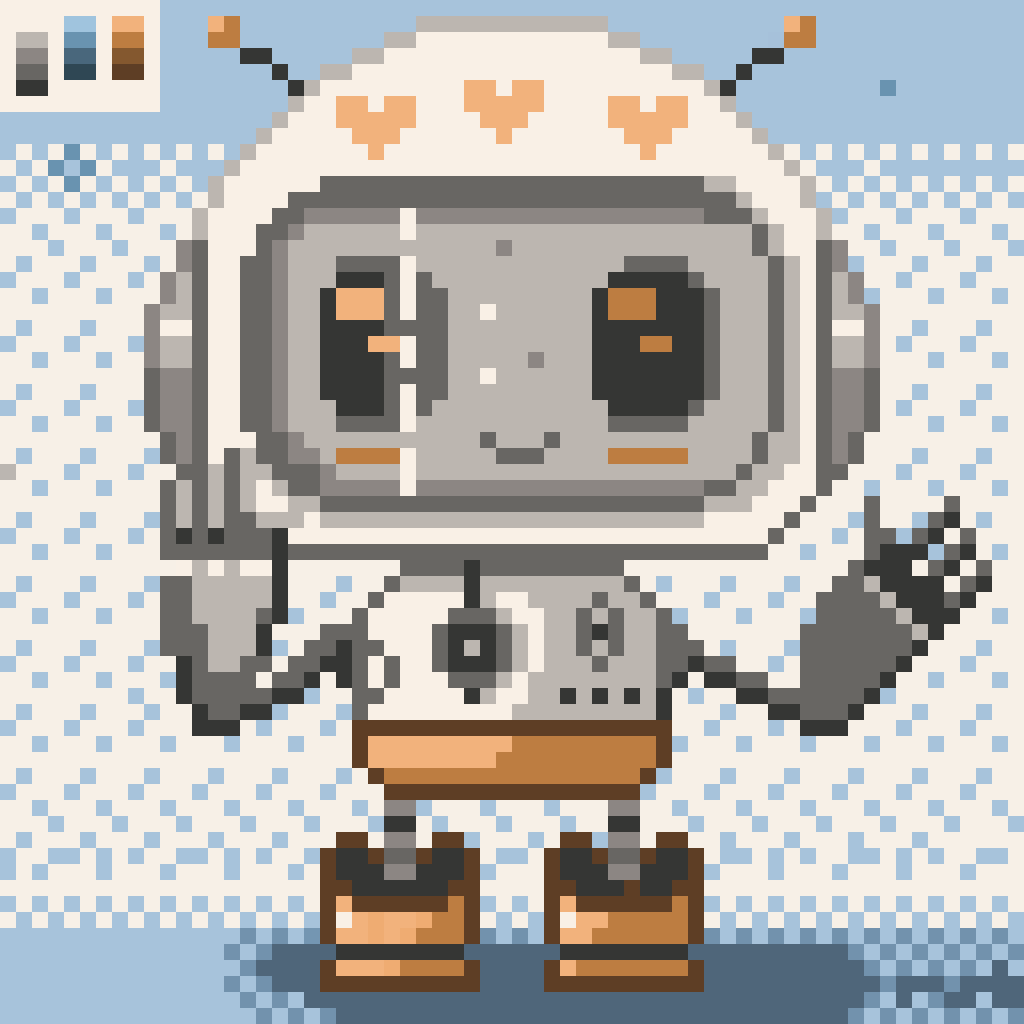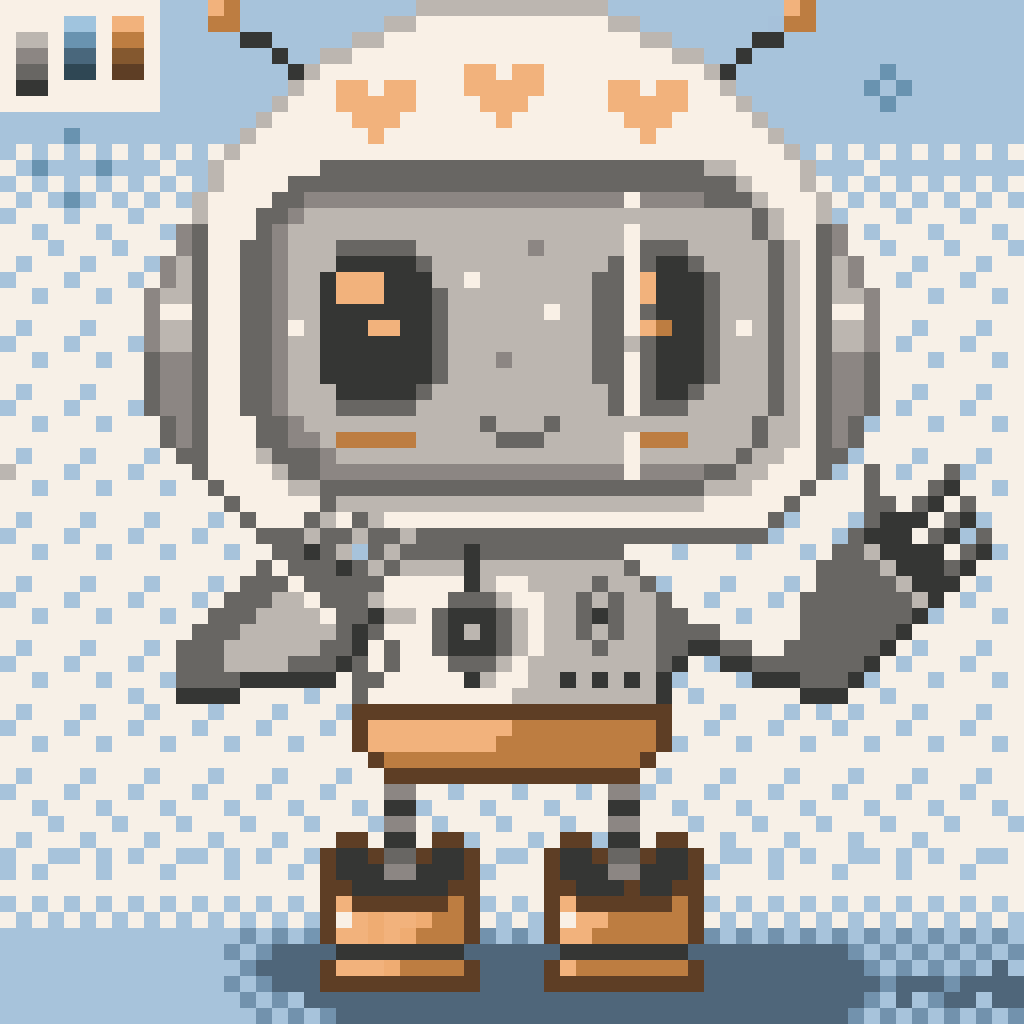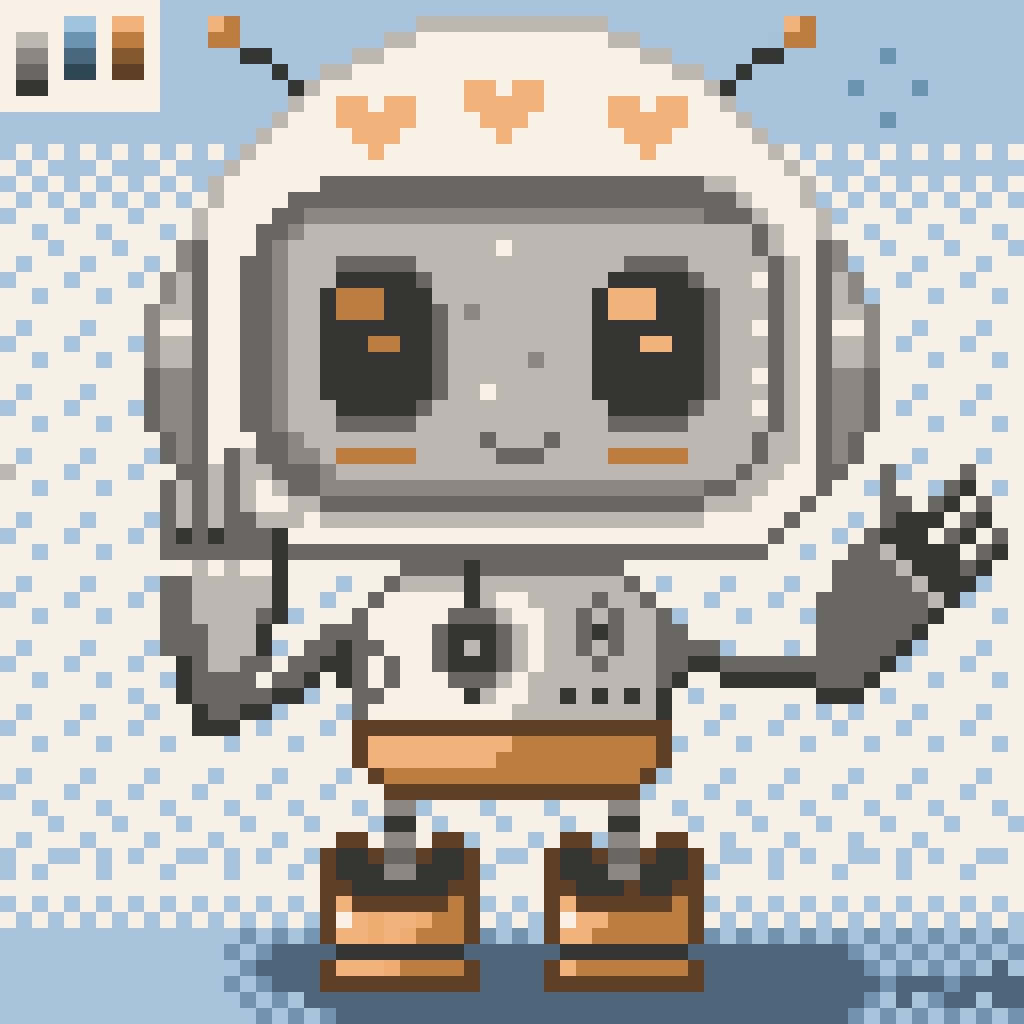
-
If you assume you’re right, you’re probably aren’t. This is the self-deprecating idea that sprouted from using the import filter of the Divoom pixel editor wrongly. I thought it needed more pixels to avoid blurring them, like web browsers. Not so, just import 92 frames or less of 64 x 64 pixels.

-
According to the robot 🤖 this is a snow leopard, which seems fair considering the coloring, but I meant to draw a tiger 🐅 Ah well, it was from memory, and hence didn’t look much like the real deal anyway.

-
In this time of glorification of autocrats it seems somewhat uncool to post a selfie, since it tends to narcissism, one of the traits of an autocrat. Nevertheless, it was a fun exercise in panning around a main subject, even if it’s me, represented in pixel art for the Pixoo64.

-
I used a reference photo—downloaded a long time ago—of a tiger walking in the snow, and thought of animating it as such. It didn’t come to that, yet, because of lack knowledge of animal anatomy. The creation started in Pixquare, refinement in Procreate, and final touches in Divoom’s pixel editor.

-
Dreaming at the dinner table when I was a preteen. We didn’t talk much, so I had to use my imagination to fill the silence. Vroooom-vroooom! And oh, it was todays theme #forklift from @pixeldailies.bksy.social #PixelDailies

-
There’s room for improvement in my little garden. After all, spring’s still one day away.

-
I made a photo of a bunch of daffodils a few days ago, and made a 192 x 64 pixel drawing today, which I turned into an infinite panning frame of 64 x 64 pixels for my Pixoo64 Cloud LED display. I also entered it in the Bloom2025 competition of the Divoom community.

Here’s the reference.

-
The @pixeldailies.bsky.social theme was #castle #PixelDailies #Pixoo64
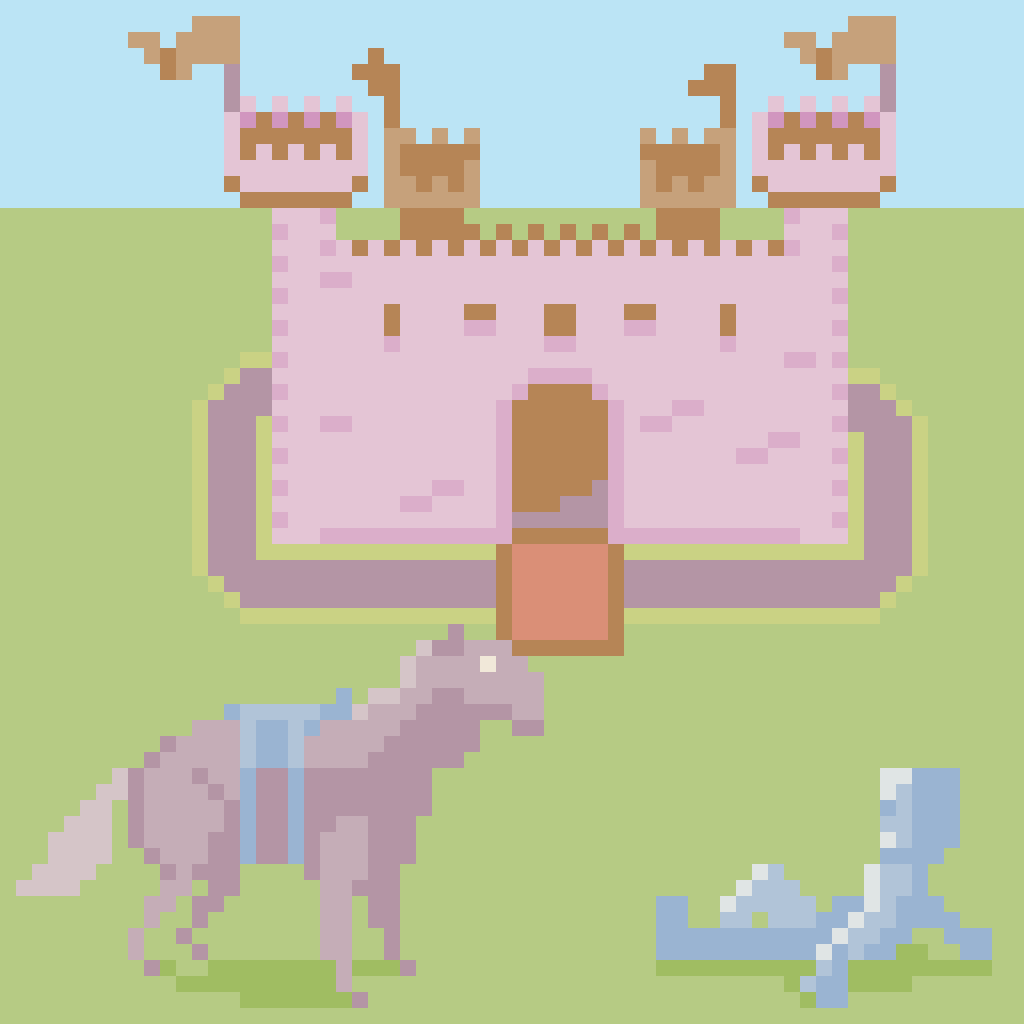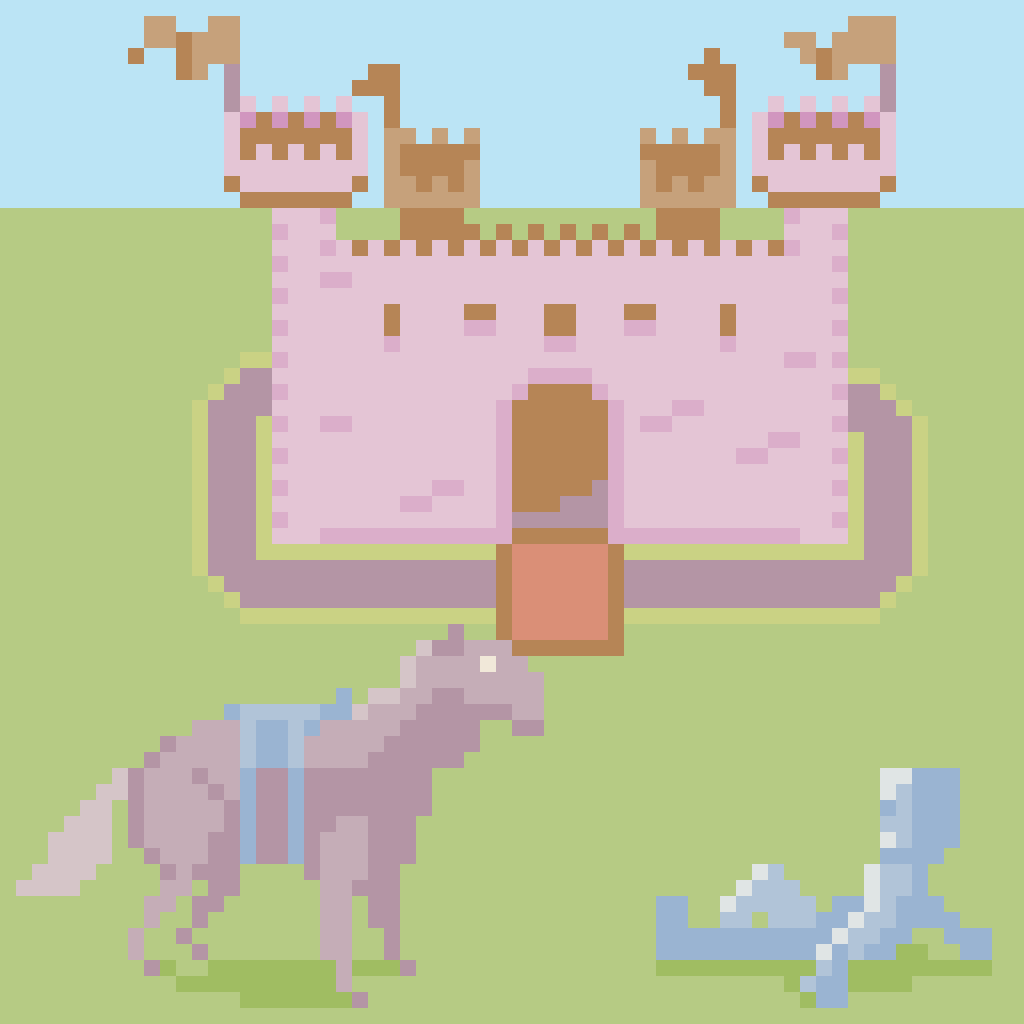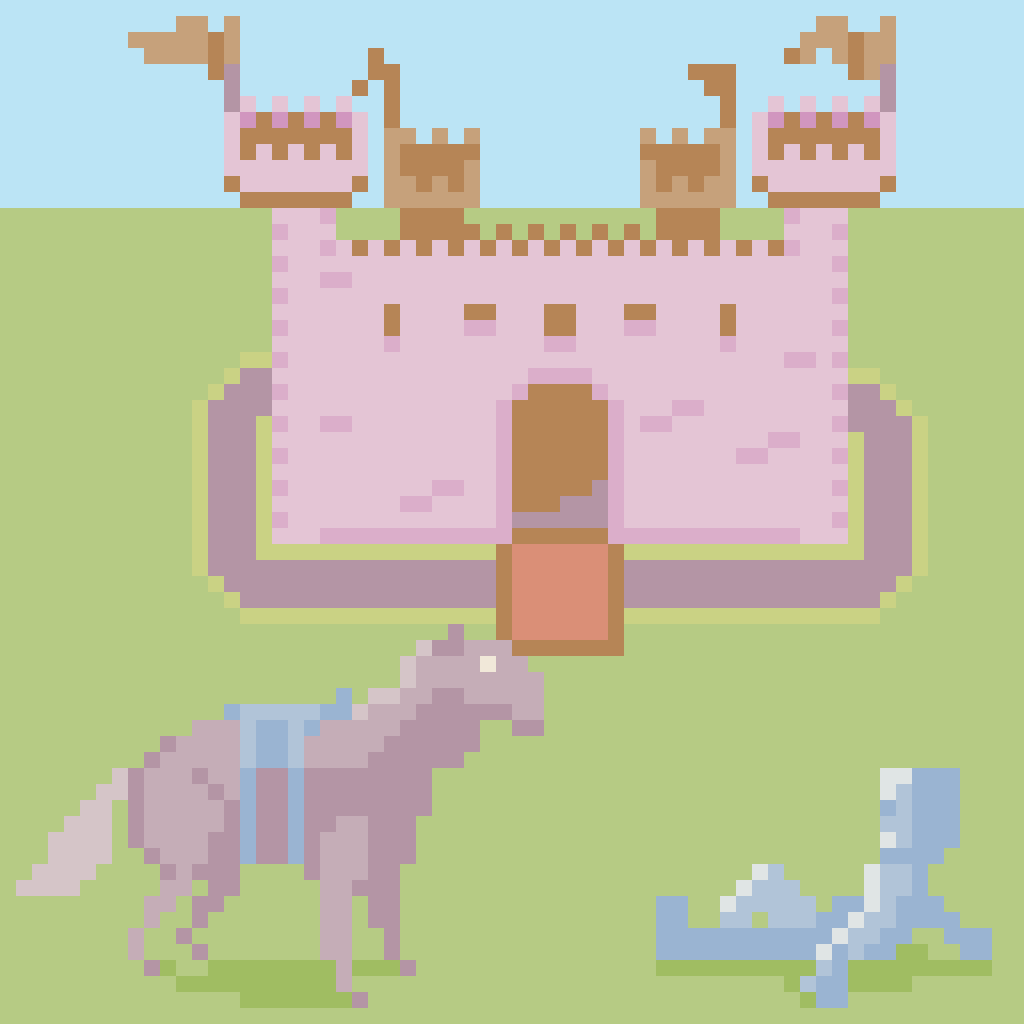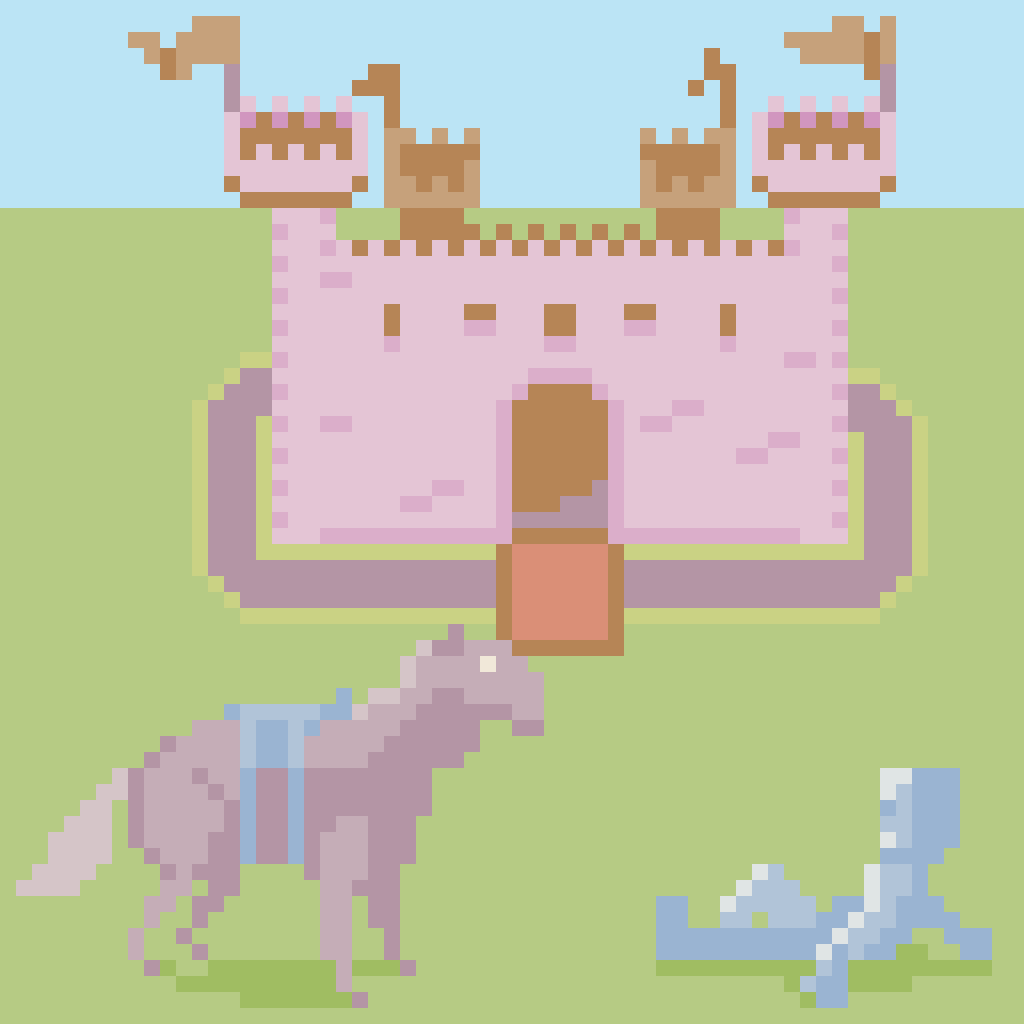
-
This was constructed for the #64x64 pixels #Pixoo64 and #PixelDailies theme #RobotController @pixeldailies.bsky.social
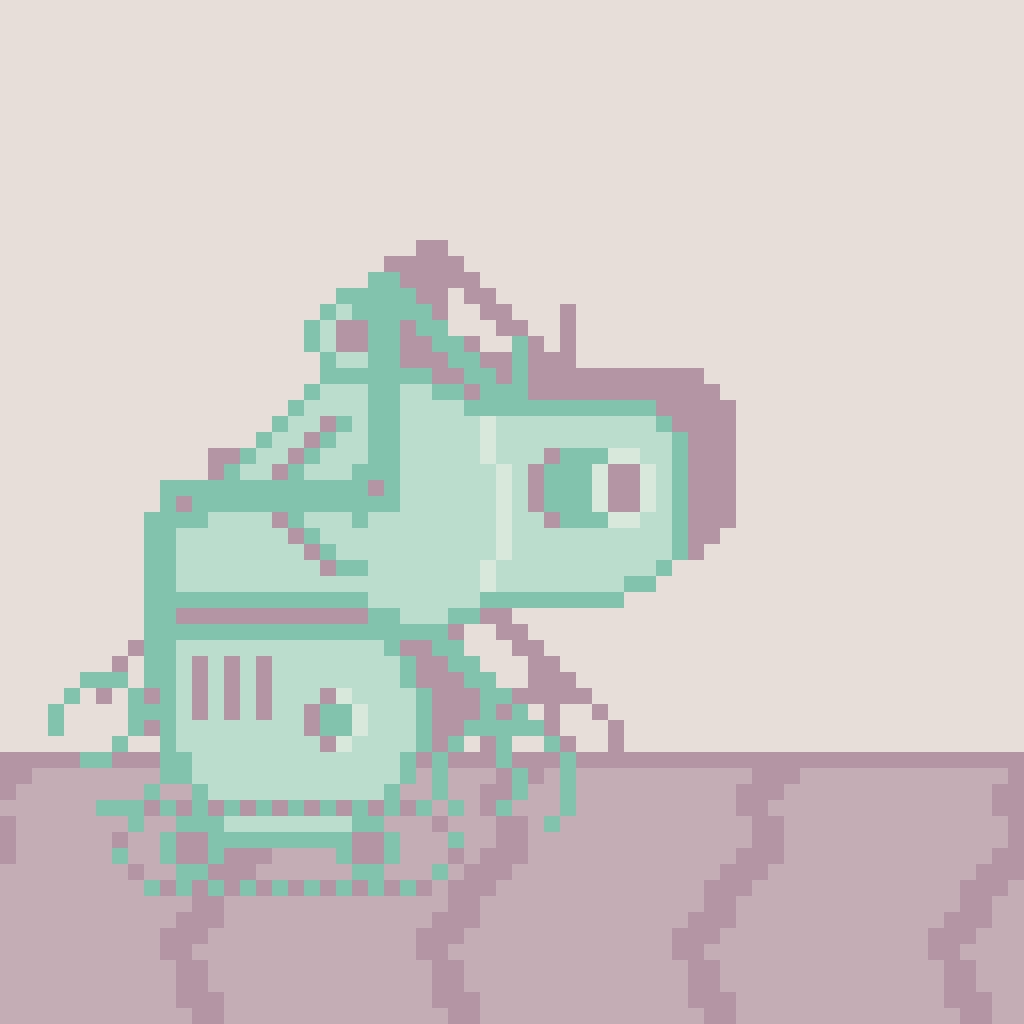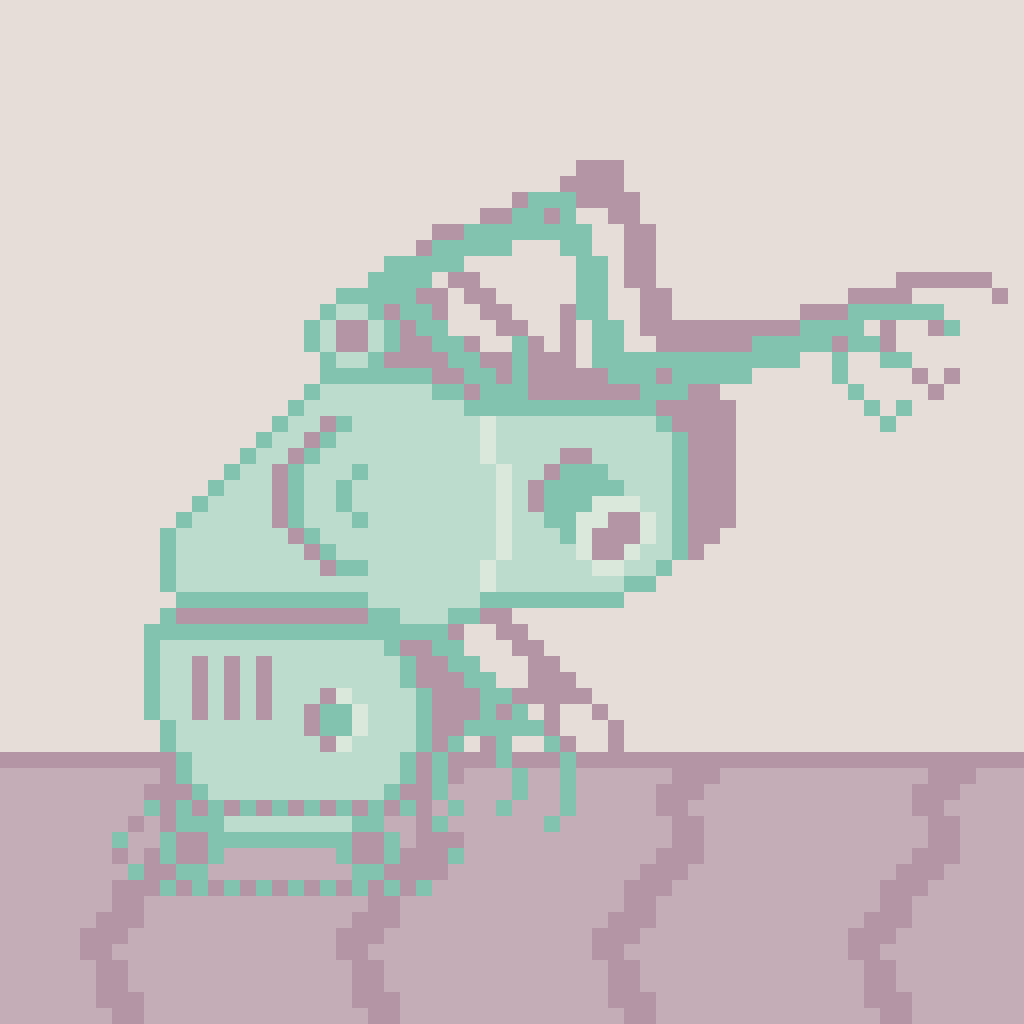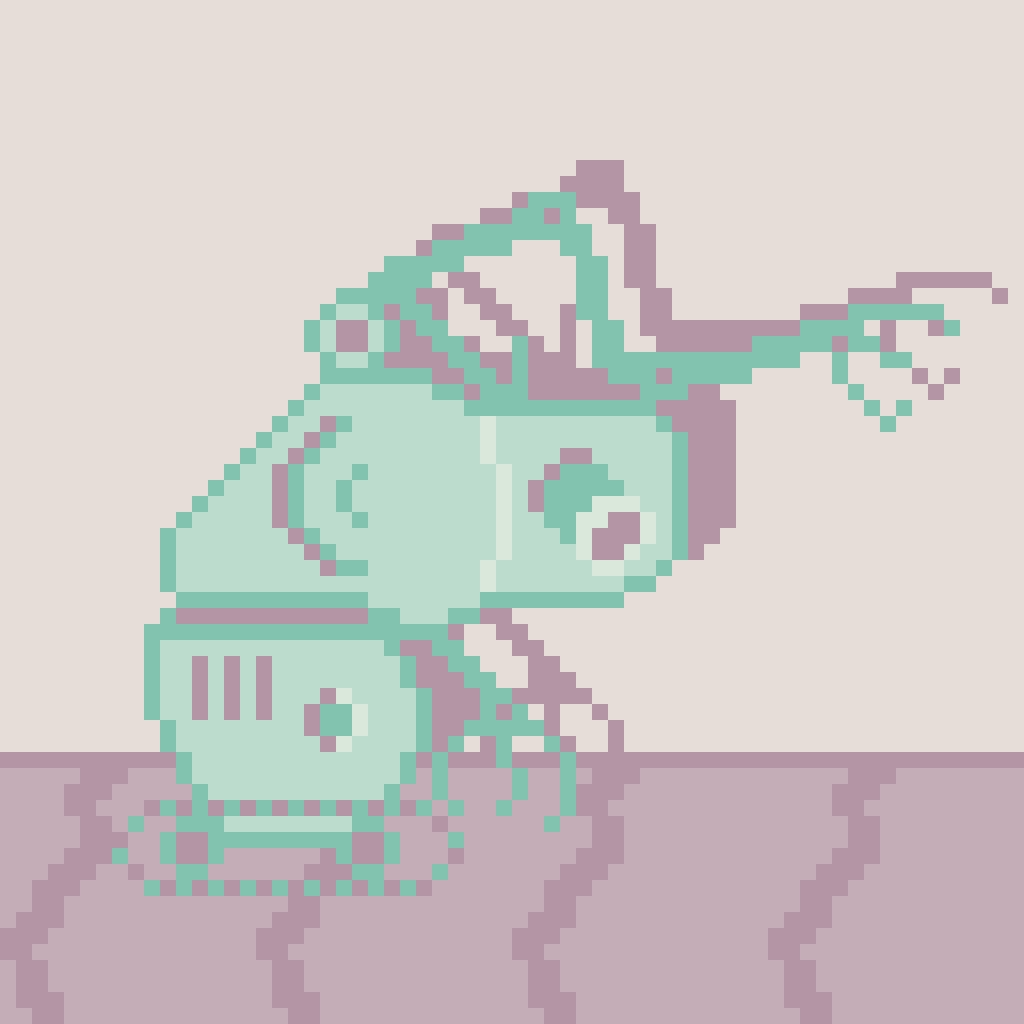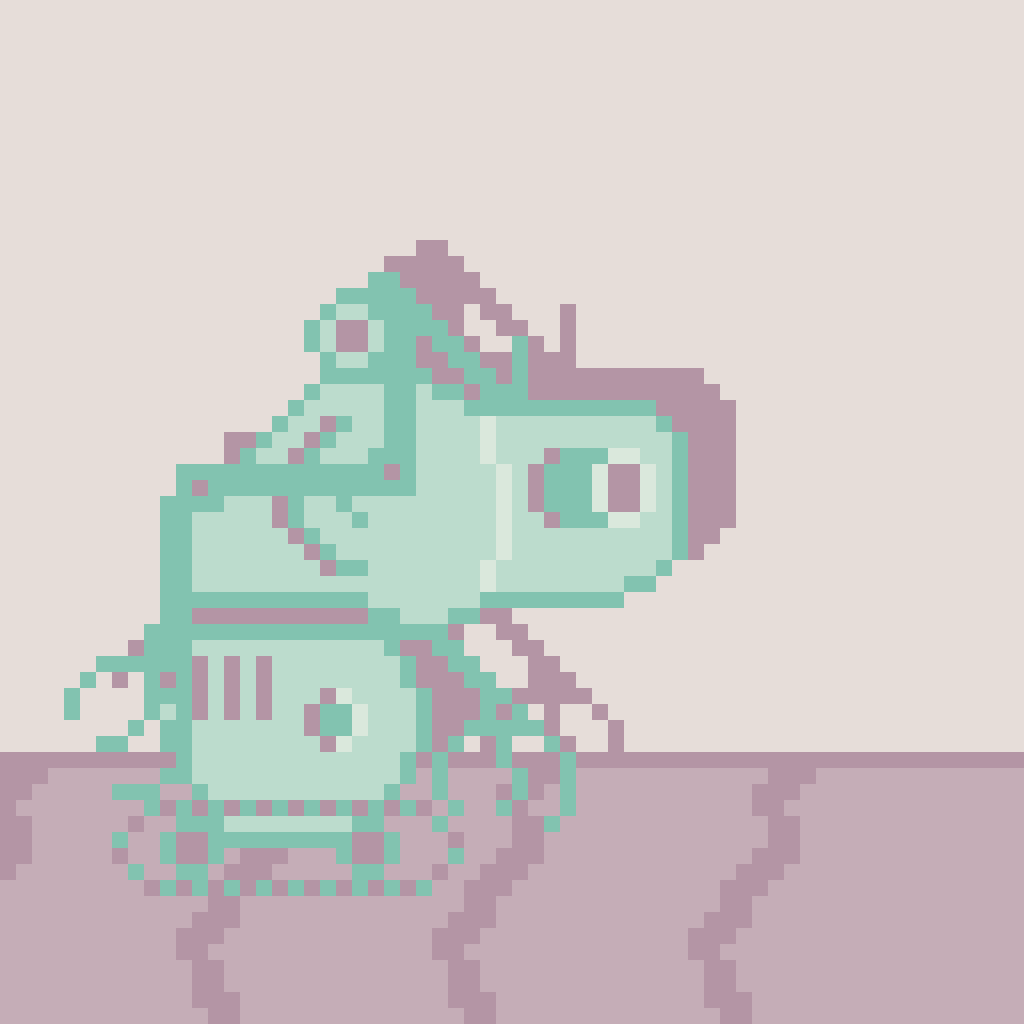
-
I made a pixel drawing of a #tiger refining it using this CC reference from Flickr. Thusly I didn’t mechanically copy the reference, and made my own version for @pixeldaily.bsky.social theme for 13 March 2025.

-
So, how should I deal with Tumblr, as it has not yet joined the Fediverse? Should I assume it joining soon, and use @ mentions to Fediverse users, or should I make an exception and link with the user’s permalink to their user profile on their prime Fediverse server?
Any help is appreciated.
-
The theme of Pixel Dailies for 12 March 2025 was #frame #64x64 #PixelDailies #Pixoo64

-
The theme of Pixel Dailies for 10 March 2025 was #OversizedClothing #64x64 #PixelDailies

-
At least the robot 🤖 got the number right, otherwise putting candles on my humongous BD cake has become ridiculous, and a real chore. 🎂

-
I really liked this video by Asianometry about heat in IC’s, and how it complicates development of chips, because how heat behaves at the quantum level is still unknown. This is one of the reasons developing nanometer scale microprocessors is so expensive and time-consuming.
-
For when I’m too busy making pixel art…